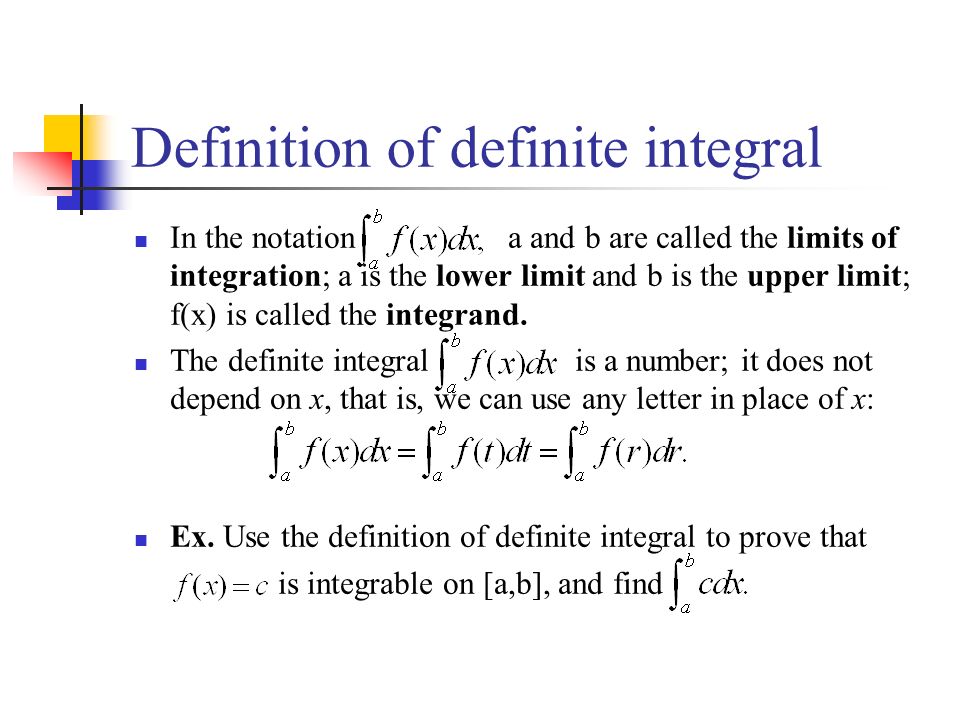
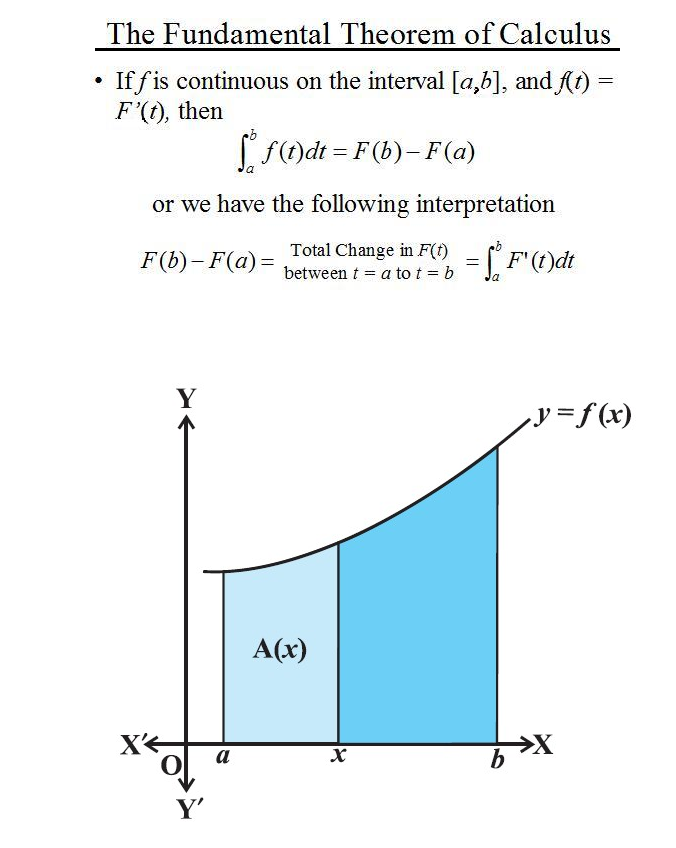
| Derivatives | Integrals (Anti Derivative ) |
|---|
| (i) `d/(dx) ( (x^(n+1))/(n+1) ) = x^n` Particularly `d/(dx)x = 1` | `int x^n dx = x^(n +1) /(n+1) + C , n != -1` ` intdx = x +C` |
| (ii) `color{blue} {d/(dx) (sinx) = cos x}` ; | `color{green} { int cos x dx = sinx + C` |
| (iii) `color{blue} {d/(dx) ( - cos x ) = sinx}` ; | `color{green} {int sinx dx = - cos x + C}` |
| (iv) `color{blue} {d/(dx) (tan x) = sec^2x}` ; | `color{green} { int sec^2 x dx = tan x +C}` |
| (v) `color{blue} {d/(dx) (-cot x ) = cosec^2x}` ; | ` color{green} { int cosec^x dx = - cot x +C} ` |
| (vi) `color{blue} { d/(dx) (sec x ) = sec x tan x }` ; | ` color{green} {int sec tan x dx = sec x + C}` |
| (vii) `color{blue} {d/(dx) (- cosec x ) = cosec x cot x }`; | `color{green} {int cosec x cot x dx = - cosec x + C} ` |
| (viii) `color{blue} {d/(dx) (sin^-1 x ) = 1/ sqrt (1 -x^2)}` | `color{green} {int (dx)/sqrt(1 -x^2) = sin^(-1) x + C }` |
| (ix) `color{blue} {d/(dx) ( - cos^(-1) x ) = 1/ sqrt (1 -x^2)}` | ` color{green} {int (dx)/sqrt(1 -x^2) = - cos^(-1) x + C}` |
| (x)` color{blue} {d/(dx) ( tan^(-1) x ) = 1/ (1 +x^2)}` | `color{green} { int (dx)/sqrt(1 +x^2) = tan^(-1) x + C}` |
| (xi) `color{blue} { d/(dx) ( - cot ^(-1) x ) = 1/ (1 +x^2)}` | `color{green} {int (dx)/sqrt(1 +x^2) = -cot^(-1) x + C}` |
| (xiii) `color{blue} {d/(dx) (sec^-1 x) = 1/(x sqrt(x^2 -1))}` ; | `color{green} { int (dx)/(xsqrt(x^2 -1) ) = sec^-1 x +C}` |
| (xiii) `color{blue} {d/(dx) ( - cosec^-1 x) = 1/(x sqrt(x^2 -1))}` ; | `color{green} { int (dx)/(xsqrt(x^2 -1) ) = -cosec^-1 x +C}` |
| (xiv) `color{blue} {g/(dx) (e^x) = e^x}` ; | `color{green} {int e^x dx = e^x +C}` |
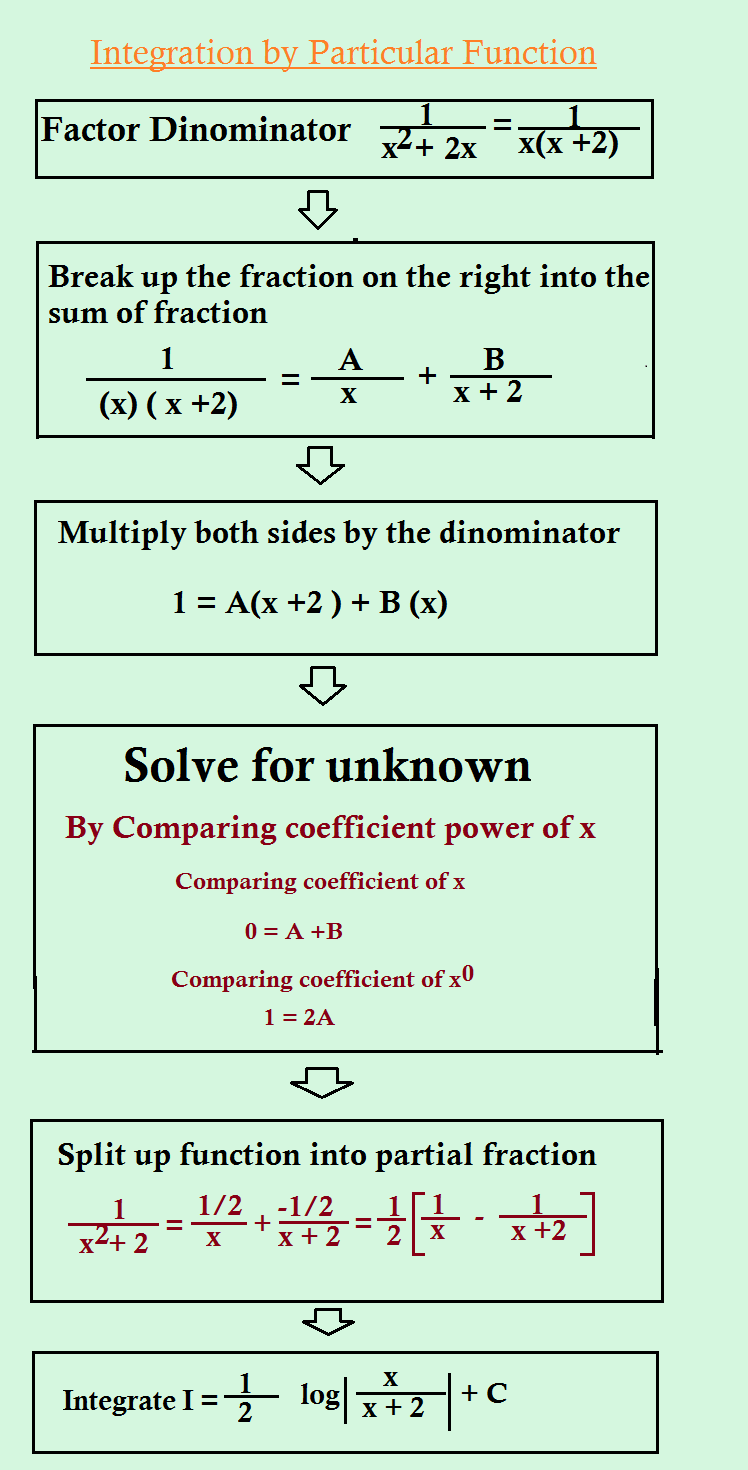
| (xv) `color{blue} {d/(dx) ( log | x | ) = 1/x}` ; | ` color{green} {int 1/x dx = log |x| +C}` |
| (xvi) `color{blue} {d/(dx) ( a^x/ (log a) ) = a^x}` ; | `color{green} {int a^x dx = a^x/(log a ) +C}` |
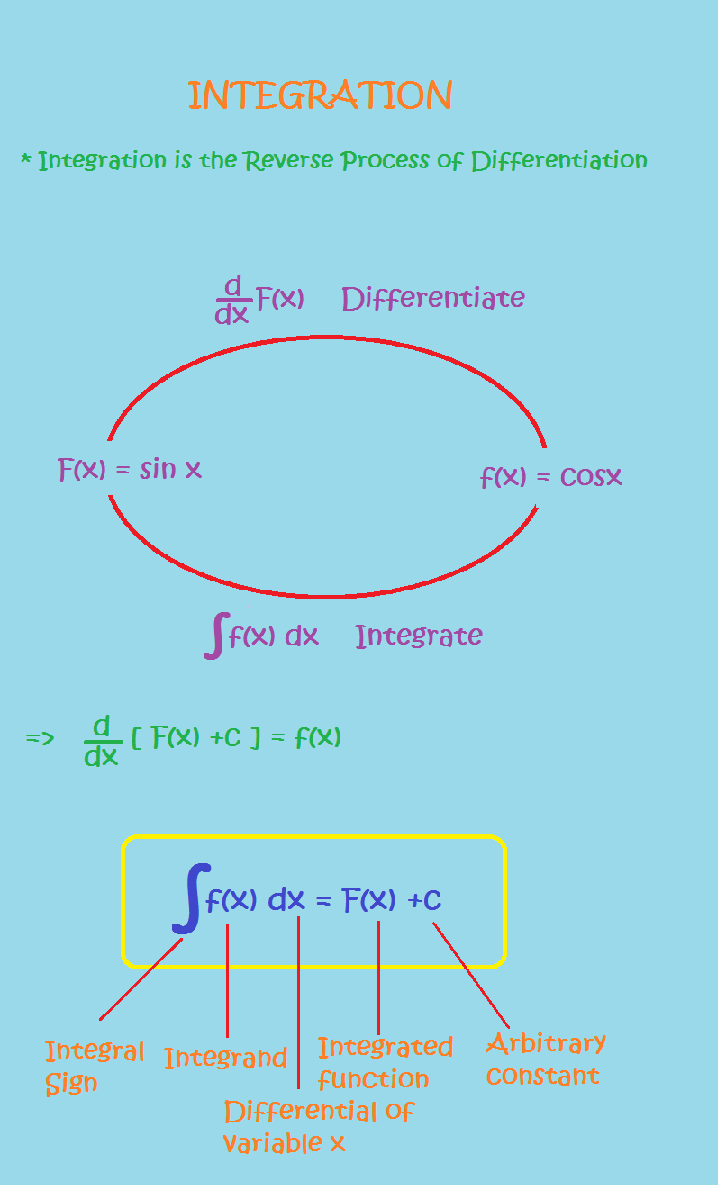
.png)
.png)
.png)
.png)
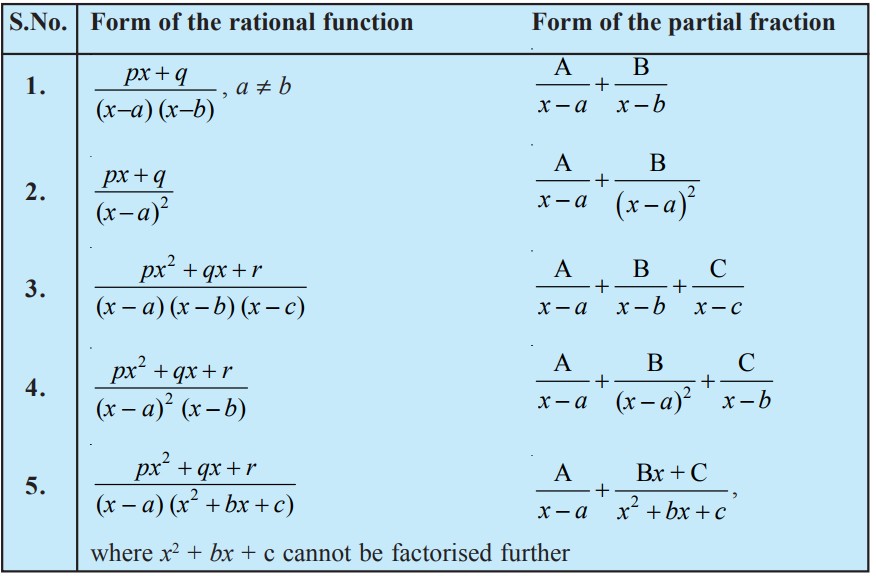
.png)